Final Project

Jonathan Beal

Bouncing Barney Brokers Bad Brew in the Back of the Black Buick.

In this project, we are going to explore triangles and paths.

Bouncing Barney, our hero, starts from one spot and travels around, but only by following a parallel path to one of the sides of the triangle.

He begins at a point in, on, or outside a triangle.

1) He travels parallel to the right arm of the triangle.

2) Next, Barney follows along side of the base of the triangle.

3) Then he walks parallel to the left side of the triangle.

4) Repeat.

The neat trick to Barney is that he always ends up back where he started.

Click here to see a GSP file of Barney. Slide the starting points back and forth.

How do we know for sure that he will always get back to his starting point? From the GSP illustration, it looks as if from any triangle, he will always end up home.

But I need to prove that Barney will always get back to where he started.

I want to look at how far Barney is travelling? There are different cases that we need to investigate.

1) If Barney starts at the centroid, where the medians meet, then ...

Since the centroid is concurrent with the parallel lines of the sides of the triangle, then Barney would easily get back to where he started.

2) If Barney starts at the midpoint on any side, then...

Barney would go half the distance of the perimeter in his journey back to his starting point. Another way to look at it could be

3) What if Barney started somewhere else besides the midpoint of one of the sides of the triangle?

Notice I used a equilateral triangle to perform this task. I can show on other triangles that the path that Barney takes is equal to the perimeter.

Got Proof?

Now what happens if Barney doesn't start at an easy location like on the side of the triangle?

If Barney starts anywhere in the graph, actually, he will already be on a path that will take him back to where he started.

We could compare Barney's path to a circle; it doesn't matter where he starts, he will always make a full rotation to end where he began.

The starting point is in the interior of the triangle, but Barney is going to still move about in the same manner. Essentially, every spot we put Barney already has a predetermined path and is exactly like all the others.

Here is another example of placing Barney in the interior of the triangle. In this case, Barney is placed at the orthocenter. The orthocenter is the concurrent point where the three altitudes of the triangle meet.

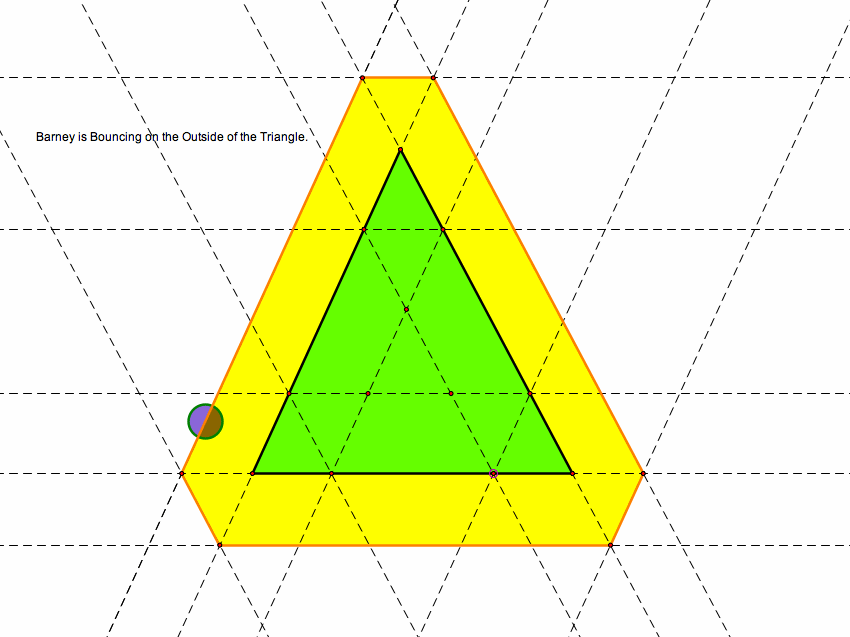
In the last exploration, I wanted to look at the path that Barney travels if he is outside of the triangle. After looking at some of the other journeys barney has taken, I noticed an inner triangle that could represent the original triangle.

My hypothesis is that, when Barney is outside the original triangle and he will perform the same way, the original triangle will become the inner triangle that I have viewed earlier.

From the proof, the parralleograms that I used to explain the path and the perimeter will be on the outside of the triangle, rather than inside.

My assumption about the construction of the paths was correct. We have been creating a similar image every time we explore Barney on a path. The outer triangular image will expand due to how far away Barney is from the original triangle. We could shrink the first triangle, have Barney follow the same path, and he will always come back. Use the GSP illustration for Barney's path around the outside of the triangle.

Go back to Home Page